1 Introduction
In this document, we construct a framework within which we can construct the portfolio of the Polar Star Overlay fund. The overlay fund can invest in the Polar Star Limited, Polar Star Spectrum, and Polar Star Quantitative Commodity funds. We have a monthly return series for Polar Star Limited from July 2010. We use backtested data for Polar Star Quantitative from July 2010 together with live data from June 2018. For Polar Star Spectrum we have to extend the monthly returns series using the Barak Shanta monthly returns. Here we have data from March 2014 to Feb 2018 followed by a gap. The returns series starts back up again in August 2018 and continues to the most recent monthly returns.
A prerequisite for the management of the overlay fund is the ability to change allocations to the underlying funds depending on how we view the available opportunities and the confidence each manager has in his view. This prerequisite immediately brings the Black-Litterman model to mind and more specifically the extension to the original model by Idzorek. This extension of the original work tilts the allocations toward those views with higher confidence in the expected returns.
2 Baseline Weights
To apply the Black-Litterman we require a baseline allocation to the three funds. In order to obtain baseline weights, we perform a risk-adjusted return portfolio optimisation with the returns stream of the three funds. We are interested in the out of sample weights of the optimised portfolio. We use a training period of 12 months from which we determine the optimal portfolio in the following month. The time window is then expanded forward by and month and the process is repeated. The evolution of the weights is shown in the plot below.
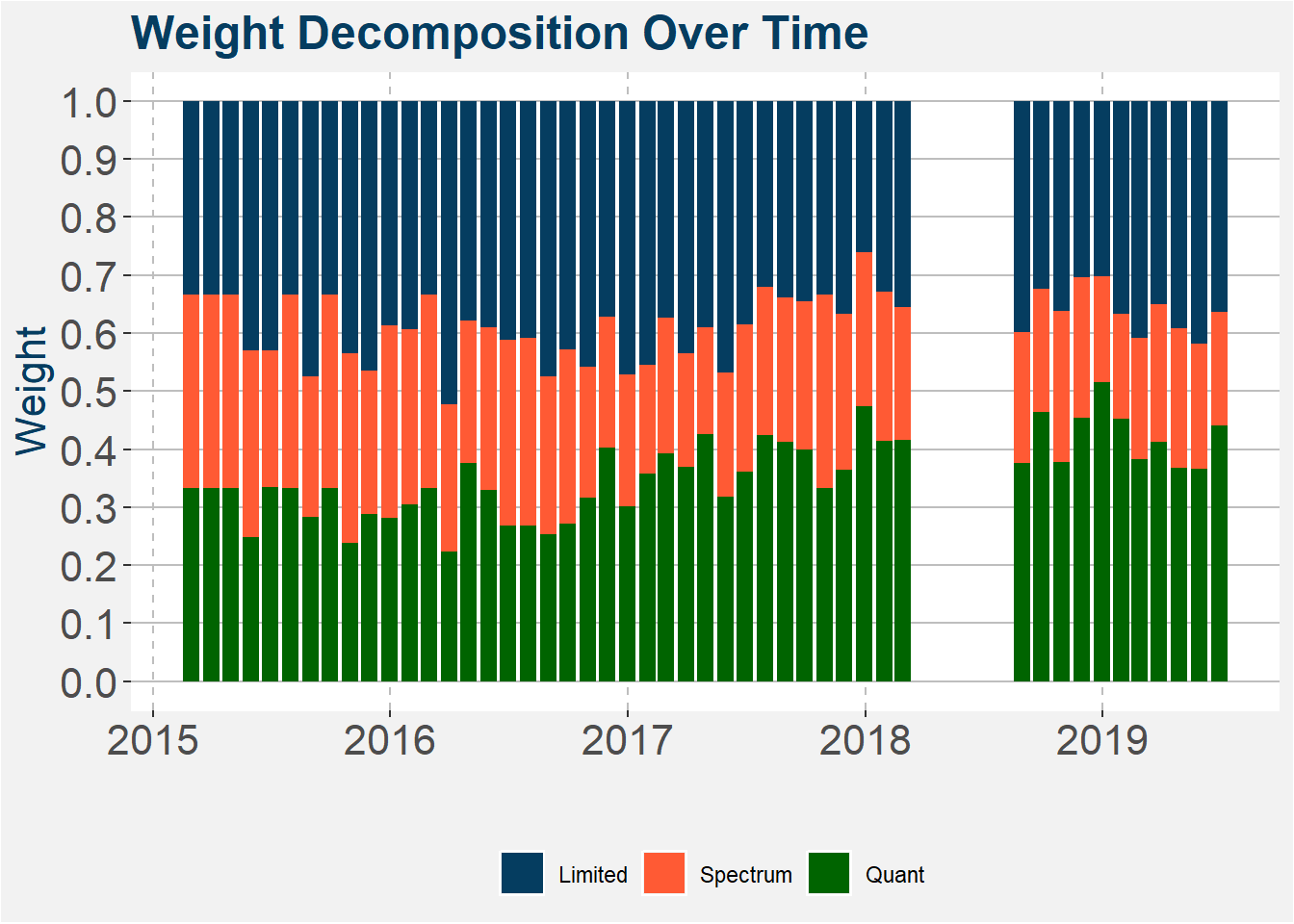
We believe that to some extent the past will repeat itself in the future. However, we are not sure which part of the past will repeat itself. For this reason, we make the assumption that all past histories are equally likely and use the average weight allocation of all the optimised time windows. The weights are given in the table below.
| fund | weight |
|---|---|
| Limited | 0.385 |
| Spectrum | 0.260 |
| Quant | 0.355 |
We define these weights as our baseline weights. Projected returns and confidence in the returns will be used to tilt away from this baseline.
3 Effect of confidence on weights
If you are interested in the mathematical details of the view and confidence extension to the Black-Litterman model by Idzorek please follow the link. We will not go through any of the details here. Instead, we will investigate the effect of changing confidence in the positive return forecast.
As a starting point, we show the statistics of months with positive returns in the table below. We use the average positive return as our expected return. The Percentage of positive months we use as a proxy for confidence in the expected return.
| statistic | Polar Star Ltd | Polar Star Quant | Polar Star Spectrum |
|---|---|---|---|
| count | 59.0000000 | 127.0000000 | 30.0000000 |
| mean | 3.8790424 | 3.8935599 | 4.7289184 |
| std | 3.0235055 | 3.6879217 | 4.0882991 |
| min | 0.2258288 | 0.1358974 | 0.1500000 |
| 25% | 1.5338260 | 1.4208538 | 1.5750000 |
| 50% | 3.3752929 | 2.7603708 | 3.1800000 |
| 75% | 5.7573100 | 5.3382040 | 7.2800000 |
| max | 11.7731761 | 21.0665602 | 14.7400000 |
| number of months | 101.0000000 | 227.0000000 | 52.0000000 |
| Positve Percentage | 0.5841584 | 0.5594714 | 0.5769231 |
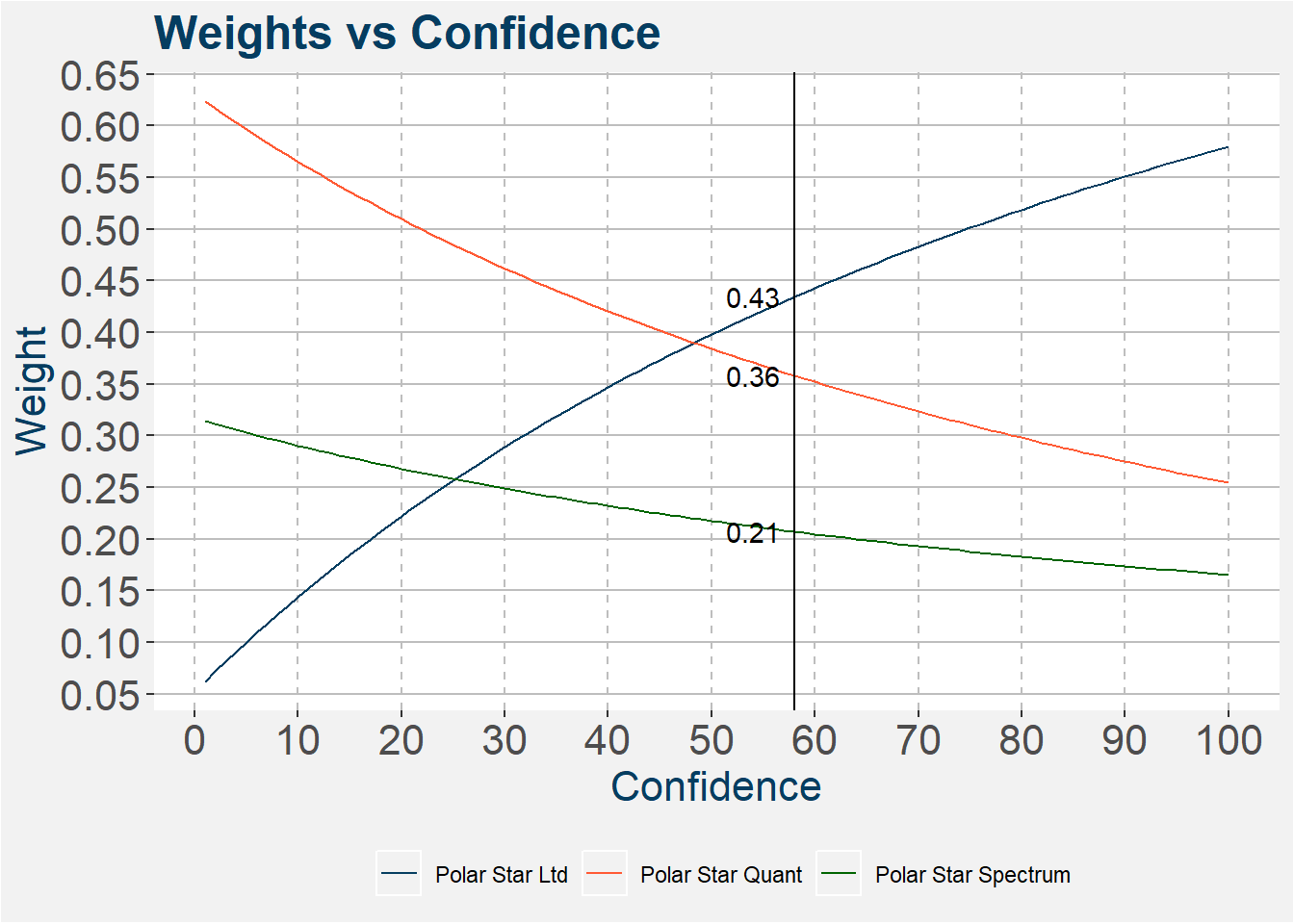
We fix the confidences of Polar Star Quantitative and Polar Star Spectrum to their values as indicated in the table above. Next, we vary the confidence of the expected return on Polar Star Limited from 1% to 100% and calculate how the weight changes between the three funds. The results are shown in the table below. The vertical black line shows the baseline confidence as given in the table above. The weights indicated next to the vertical line show the weights at the baseline numbers. Notice that the allocation to Polar Star Limited increases as the confidence in the expected return increases.
4 Conclusion
Using the Black-Litterman model together with the extension by Idzorek we can create a systematic framework that uses a discretionary input to tilt the allocation according to the confidences in expected returns. This serves as a starting block for the allocation of the Polar Star View fund.